|
1.
Where such combination is possible, sketch the initial arrangement
of AO's and final bonding and antibonding MO's for the combination
of the following AO's into MO's: (a) two s AO's into two s-
MO's (b) two p AO's into two p MO's
(6) two s AO's into two p MO's (d) two
p AO's into two s- MO's (e) an s and
a p AO into two s- MO's (f) an s and
a p AO into two p MO's For the impossible
combinations, explain why they are impossible.
2. Add the nodes, or surfaces of zero electron probability,
to the sketches of Problem 1. What relationship is there between
the number of nodes in the bonding and antibonding orbitals from
the same two AO's? How does this arise in the way the AO's are combined?
How is this correlated with relative energies?
3. If q is the angular coordinate
around the H-C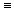 C-H
molecular axis in acetylene, then the p C-H
molecular axis in acetylene, then the p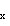 wave functions (not the orbitals) have an angular dependence given
by y(q)
= sin q, and the p
wave functions (not the orbitals) have an angular dependence given
by y(q)
= sin q, and the p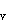 wave functions have an angular dependence y(q)
= cos q. What then is the expression
for the angular dependence of the P
wave functions have an angular dependence y(q)
= cos q. What then is the expression
for the angular dependence of the P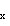 and p
and p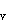 atomic
orbitals? Plot the two wave functions, and the two atomic orbitals,
on separate polar graphs and compare them. atomic
orbitals? Plot the two wave functions, and the two atomic orbitals,
on separate polar graphs and compare them.
4. In view of the angular functions given in Problem 3, prove
the statement in the text that the combined p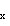 and p
and p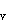 MO's in acetylene form a cylindrical barrel of electron density,
the same in all directions, q, around
the molecular axis.
MO's in acetylene form a cylindrical barrel of electron density,
the same in all directions, q, around
the molecular axis.
5. The bond energies for typical carbon-carbon single and
double bonds are 83 kcal mole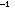 and 147 kcal mole
and 147 kcal mole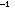 ,
respectively. Which of the following would you expect as the triple
bond energy: 106, 150, or 194 kcal mole ,
respectively. Which of the following would you expect as the triple
bond energy: 106, 150, or 194 kcal mole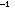 ? ?
|
|