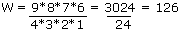How can we correct for this overcounting? How do we "remove the labels" from the atoms? As a correction factor, how many different label shufflings can be made for each arrangement of four atoms? Label �a� could be given to any one of the four atoms, label �b� to any of the remaining three, label �c� to two, and label �d� then has to go to the last atom. There are 4 * 3 * 2 * 1 = 24 meaningless permutations of labels for every really different arrangement of atoms. We have overcounted by a multiplicative factor of 24.

Hence the 3024 ways of arranging atoms must be divided by 24 to remove the labels on the atoms. The number of different ways of arranging four indistinguishable atoms among nine locations is

Of the 126 possible arrangements only four are crystals, and the other 122 lead to a gas. Even in such a tiny and restricted universe, a gas is far more likely to result from a random arrangement of atoms than is a crystal. This is true because the specifications for a crystal are so much more restrictive:

Crystal - four adjacent atoms in a square;

Gas - four atoms in any arrangement except those that lead to a crystal.

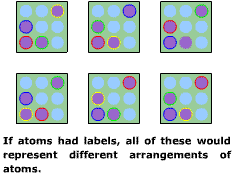
Since the atoms do not have names or labels, and all look alike, all of the above pictures correspond to the same single atomic arrangement. That is four atoms at positions 3, 4, 7, and 8.