|
However,
in the much more powerful nuclear reactions, these principles of
separate conservation of mass and energy must be combined into the
conservation of the total of mass and energy. Mass can be
converted into energy and energy into mass according to Einstein's
relationship E = mc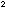 ,
in which E is energy, m is mass, and c is the
velocity of light. In the last half of this chapter we will discuss
nuclear reactions for which this mass-energy conversion is important.
The conversion of mass into energy is central to both nuclear fission
and nuclear fusion, on this planet and in the sun. ,
in which E is energy, m is mass, and c is the
velocity of light. In the last half of this chapter we will discuss
nuclear reactions for which this mass-energy conversion is important.
The conversion of mass into energy is central to both nuclear fission
and nuclear fusion, on this planet and in the sun.
In principle, if a reaction gives off energy, the products formed
must have lower energy and be lighter than the reactants.
But a release of 100 kcal 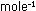 by a typical chemical reaction corresponds (via the Einstein relationship)
to a mass loss of only 5 x 10
by a typical chemical reaction corresponds (via the Einstein relationship)
to a mass loss of only 5 x 10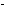  amu per molecule, or one hundred thousandth the mass of an electron.
This amounts to only 5 x 10
amu per molecule, or one hundred thousandth the mass of an electron.
This amounts to only 5 x 10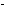  gram per mole, which is far less than we can measure. This is why
we can say that, for chemical reactions, mass and energy are conserved
independently.
gram per mole, which is far less than we can measure. This is why
we can say that, for chemical reactions, mass and energy are conserved
independently.
Many properties other than mass are not conserved in chemical reactions:
volume, density, shape, thermal conductivity, hardness, color, and
others. It was Antoine Lavoisier, the brilliant French chemist who
revolutionized chemistry before he went to the guillotine in 1794,
who realized that mass was more fundamental than any of these
properties. When you ask "How much?" in chemistry, you basically
are asking "What mass?"
|

|